
- Home >
- Raven Paradox
Raven Paradox
The Flaws in the Scientific Method
The Flaws in the Scientific Method
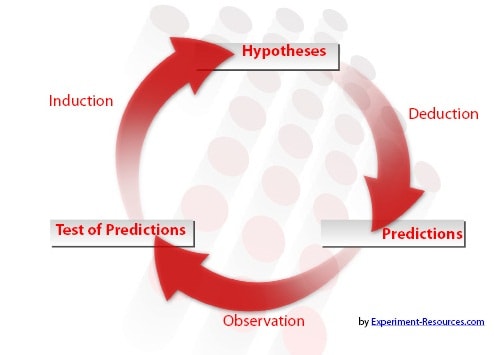
All scientists use reasoning and logic at some stage, to create hypotheses and design robust experiments.
This article is a part of the guide:
Browse Full Outline

Hempel’s Logic
In a beautiful and elegant treatise, the German philosopher Carl G Hempel, in 1965, showed that there were flaws in these long-established processes. His Raven Paradox called into question the established processes of inductive reasoning, generalization and falsifiability.
The Inductive Hypothesis
Imagine that a scientist, after years of going for long walks in the countryside, observes that every single raven he has ever seen is black.
As a dutiful researcher, he uses inductive reasoning to postulate the hypothesis:
"All ravens are black.”
This is a perfectly acceptable conditional hypothesis. Firstly, it is testable, because you could sample raven populations and verify that they are all black.
The statement is also falsifiable because even one non-black raven amongst the sampled population would disprove the hypothesis.
This is all great science, so far, following the established methods of inductive reasoning. The researcher could even design an experiment to sample raven populations, with thousands of ravens observed.
If they are all black, the hypothesis is supported and plausible. Over time, repeated experiments and observations further confirm this and the hypothesis becomes accepted as a law.
The Problem of Generalization and Falsifiability
The first part of the Raven Paradox proposal questions the process of generalization.
It is practically impossible to sample every single raven in the world and there may be a few non-black individuals. Hempel was not trying to comment upon the exact science, but as an interesting aside, about 1 in 10 000 raven eggs contain partially or fully albino birds.
Most albino birds are more visible to predators, suffer from health problems and may be a localized phenomenon. The odds of seeing an albino raven are very small and sightings are extremely rare. A researcher could sample many thousands of Ravens and not see one white bird, even though they do exist.
Thus, the notion of falsifiability is questioned and undermined by the Raven Paradox. Although the original hypothesis is technically falsifiable, in practical terms it is very difficult to disprove, because the chances of observing a white Raven are very slim.
Even if you sampled the entire known population of ravens, there may be an undiscovered group containing a non-black individual.
Flaws in the Inductive Reasoning Process
The next part of the Raven Paradox questions the processes of inductive and deduction reasoning that are an integral part of the scientific process.
When a researcher states that all ravens are black, the laws of logic demand that this conditional statement has a contrapositive, statement.
Therefore, according to inductive reasoning, “Everything that is not black is not a raven.” This means that every non-black object observed, that is not a raven, equally strengthens the hypothesis. There are a countless number of non-black objects in the universe and we should pity the poor statistician who has to analyze this!
To take the analogy further, another researcher in another part of world, through fluke, may have only seen one raven in their life, which happened to be white. Their deduced hypothesis may be that “All ravens are white.” Every non-white object, which is not a raven, strengthens this opposing hypothesis too. This is the Raven Paradox.
The End of the Scientific Process?
What does this paradox mean? Has the world of science come crashing down around our ears?
The answer is a resounding NO!
The Raven Paradox is a useful philosophical observation and helps to ensure that we constantly probe and test the steps of the established scientific processes.
The examples given in the paradox are simplistic and unlikely, merely serving as an exercise to test the boundaries of the philosophy of science.
In reality, for the vast majority of cases, Hempel’s treatise makes no difference and the normal reasoning and experimental design processes work perfectly well.
The paradox does not detract from science, but actually enhances it, by preventing scientists from believing that they have proved something beyond doubt.
The Raven Paradox should remind every scientist of the dangers of generalization and that they must ensure that all hypotheses are realistically falsifiable. If the researcher has said, “All ravens in Norway are black”, this is more realistic as ornithologists could feasibly observe every raven in Norway.
Paradigm Shifts
Even long-standing theories, which became established as laws and immovable paradigms, can be proved incorrect over time.
Science is really all about testing probabilities and assumption. If something has a 99% chance of being correct then it should be accepted as the likely explanation.
The chances of someone seeing only one raven in their life, which happens to be white, are tiny.
However, this is not the same as impossible and that possibility must never be ignored. This is why all experiments are rigorously validated and reviewed before gaining widespread acceptance, to minimize the effects of the Raven Paradox.
For example, Newton’s laws were accepted as truth until Einstein’s theories blew them out of the water.
In turn, General Relativity is not the answer to fundamental physics and has been superseded by other theories.
This is how science evolves, by challenging and adapting established paradigms and laws. The creation of Chaos Theory was a perfect example of ‘maverick’ scientists chipping away at the established laws until the theory could no longer be ignored. It eventually burst into the public consciousness and fractal models appeared as prints on T-Shirts.
Hempel’s Raven Paradox stands to remind us all that no theory, however established, should be immune to challenge or debate. As new evidence is uncovered, science must adapt and change to assimilate the new data.
Martyn Shuttleworth (Jul 23, 2008). Raven Paradox. Retrieved Oct 15, 2025 from Explorable.com: https://explorable.com/raven-paradox
You Are Allowed To Copy The Text
The text in this article is licensed under the Creative Commons-License Attribution 4.0 International (CC BY 4.0).
This means you're free to copy, share and adapt any parts (or all) of the text in the article, as long as you give appropriate credit and provide a link/reference to this page.
That is it. You don't need our permission to copy the article; just include a link/reference back to this page. You can use it freely (with some kind of link), and we're also okay with people reprinting in publications like books, blogs, newsletters, course-material, papers, wikipedia and presentations (with clear attribution).
This article is a part of the guide:
Browse Full Outline
Footer bottom



